Fractalissimo

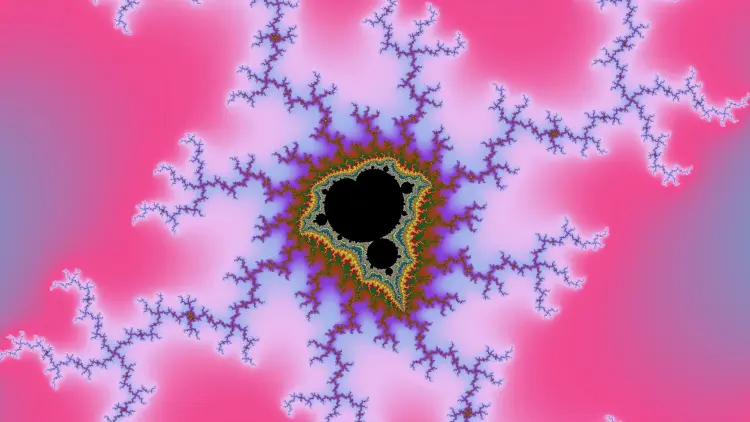
For a long time I thought of the Mandelbrot fractal as a slightly academic point - something about Romanescu cauliflowers. But today I discovered an app called Fraktal that changed all that. I'd seen some videos on YouTube, that play chill music whilst zooming in through the Mandelbrot over an hour or more. The psychedelic beauty of the patterns is breathtaking, so in order to find out how the videos were made I was led to the Fraktal app. This app creates a basic Mandelbrot pic, and allows you to zoom in, wherever you like. Unlike previous apps I'd tried, this allowed infinite zoom, at a high-speed of refresh. After zooming in a little, you begin to feel that you're possibly visiting a part of the fractal never visited before by anyone else. It's like exploring a whole new universe of pattern and colour.

The mathematics of the Mandelbrot fractal involves iterations of a tiny equation, containing complex numbers. The set is generated by iteratively applying this equation, and the visual representation is obtained by assigning colours to points based on how quickly or slowly they escape to infinity. Points that do not escape within the maximum iteration limit are often given a particular colour, usually black.

The Mandelbrot set is a self-similar fractal, meaning that it looks similar at all scales. It was first discovered by Benoit Mandelbrot in the 1970s. He was studying the behaviour of complex dynamical systems when he noticed the unusual properties of the set that now bears his name.

Mathematicians, biologists, and artists have all been amazed by the infinite power of the Mandelbrot fractal. It is evidence of the ability of mathematics to unveil the underlying complexity and beauty of the natural world.